Lines in the Plane and Incidence

We will develop the theory of lines in the projective plane $I\!\!P^{2}$. Most of the results can also be extended to higher dimensions.

Let $M= (x,y,t)^\top$ and $N=(u,v,w)^\top$ be homogeneous representatives for two distinct points in the plane (i.e. M and N are linearly independent 3-vectors: $M\not=\lambda N$). Let L=(a,b,c) be a the dual coordinates of a line (hyperplane) in the plane. By the definition of a hyperplane, M lies on L if and only if the dot product $L\cdot M$ vanishes, i.e. if and only if the 3-vector L is orthogonal to the 3-vector M. The line MN through M and N must be represented by a 3-vector L orthogonal to both Mand N, and hence proportional to the cross product $M\times N$:

$$L\sim M\times N = \left(\begin{array}{c} yw-tv\\ tu-xw\\ xv-yu \end{array}\right)$$

Since $\times$ is bilinear, the mapping $M\rightarrow MN$ for fixed Nis a linear mapping defined by the matrix

$$\left( \begin{array}{ccc} 0 & w & -v\\ -w & 0 & u\\ v & -u & 0 \end{array} \right)$$

The vector N generates the kernel of this mapping.

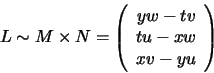
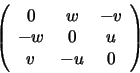
Another way to characterize the line MN is as the set of points $P=\lambda M + \mu N$ for arbitrary $\lambda,\mu$. Evidently

$$\begin{eqnarray*}(M\times N)\cdot P &=& \lambda (M\times N)\cdot M + \mu (M\times N)\cdot N \\ &=& 0 + 0 = 0 \end{eqnarray*}$$

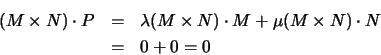
Dually, if L and L' are two lines defined by their dual coordinates, then $\lambda L + \mu L'$ is some other line through the intersection X of L and L' (since $L\cdot X=0=L'\cdot X$ implies $(\lambda L + \mu L')\cdot X=0$). As $\lambda:\mu$ varies, this line traces out the entire pencil of lines through X. By duality, $X=L\times L'$.

One further way to approach lines is to recall that if M, N and Pare collinear, each point is a linear combination of the two others. In particular, the $3\times3$ determinant |MNP| vanishes. If M and Nare fixed, this provides us with a linear constraint that P must satisfy if it is to lie on the line: $\vert MNP\vert=(M\times N)\cdot P=0$.