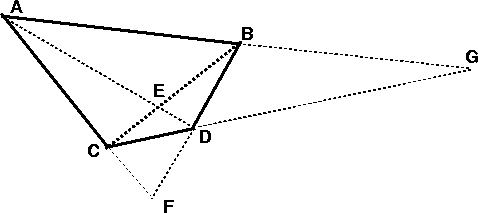
 |
Any set of four non-aligned points A,B,C,D in the plane can be joined
pairwise by six distinct lines. This figure is called the complete
quadrangle. The intersections of opposite sides (including the two diagonals)
produce three further points E,F,G (see fig. 3.4).
Property: The pencil of four lines based at each intersection of opposite sides is harmonic. For example
A simple proof maps the quadrangle projectively onto a rectangle,
sending F and G to points at infinity in two orthogonal directions.
The four lines of the pencil become parallel, with one being at
infinity. The line through E is obviously half way between those
through A and B, so by the previous exercise the line configuration
is harmonic.
![]()