Cross Ratios and Projective Bases:

In section 2.1 we saw that any 3 distinct points on the projective line can be used as a projective basis for it. (NB: if we also specify their homogeneous scales, only two are needed, as with M and N above). The cross ratio $k = \{A, B; C, D\}$ of any fourth point D with the points of a projective basis A, B, Cdefines D uniquely with respect to the basis. Rescaling to $\mu_i=1$ as above, we have

$$\lambda_4 = \frac{(\lambda_1-\lambda_3)\lambda_2+(\lambda_3-... ...2)\lambda_1 k} {(\lambda_1-\lambda_3)+(\lambda_3-\lambda_2)k}$$ (3.2)

As this is invariant under projective transformations, the cross ratio can be used to invariantly position a point with respect to a projective basis on a line. A direct application is reconstruction of points on a 3D line using measured image cross ratios.

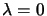
Exercise 3.4   : In an image, we see three equally spaced trees in a line. Explain how the position of a fourth tree in the sequence can be predicted. If the first tree lies at $\lambda=0$ on the image line, the second lies at 1and the third at c, what is the image coordinate of the fourth tree?